DDE-BIFTOOL demo 1 - Neuron, simplification using utilities

This demo repeats the illustrative example using the convenience functions in ddebiftool_utilities. The system of delay differential equations is [Shay,99] again

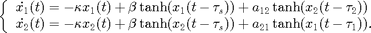
$\left\{\begin{array}{l}\dot{x_1}(t)= -\kappa x_1(t)+\beta \tanh(x_1(t-\tau_s))+a_{12}\tanh(x_2(t-\tau_2)) \\ \dot{x_2}(t)=-\kappa x_2(t)+\beta \tanh(x_2(t-\tau_s))+a_{21}\tanh(x_1(t-\tau_1)). \end{array}\right.$

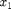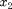This system models two coupled neurons with time delayed connections. It has two components ( $x_1$ and $x_2$ ), three delays ( $\tau_1$ , $\tau_2$ and $\tau_s$ ), and four other parameters ( $\kappa$ , $\beta$ , $a_{12}$ and $a_{21}$ ).

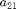The demo will show (with fewer explanations than demo1_funcs.html and its follow-on files)

continuation of equilibria in $a_{21}$ ,
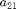computation of their stability (eigenvalues of linearization),
continuation of Hopf bifurcations in 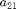$a_{21}$ and $\tau_s$ ,
branching off from a Hopf bifurcation to continue a family of periodic orbits in $a_{21}$ ,
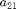computation of stability of periodic orbits (Floquet multipliers of linearization),
continuation of folds of periodic orbits in 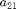$a_{21}$ and $\tau_s$

Additional Folder for Path
Definition of right-hand side
Steady state branches
Stability along branch
Hopf continuation
Figure: Continuation (predictions and corrections) of Hopf bifurcation
Linear stability along Hopf curve
Switch to second Hopf curve near double Hopf point
Figure: Continuation (predictions and corrections) of both Hopf bifurcations
First Lyapunov coefficient L1 along both Hopf curves
Determine normal form of double Hopf point
Two-parameter bifurcation diagram of Hopf bifurcations
Periodic orbit continuation
Figure: family of periodic orbits
Floquet multipliers of periodic orbits
Continuation of folds of periodic orbits
Add fold of periodic orbits to Two-parameter bifurcation diagram
save data:

Additional Folder for Path

The utility functions are stored in a separate folder, which has to be loaded in addition to ddebiftool. We also include the folders for periodic orbits and for normal form functions.

addpath('../../ddebiftool/',...
    '../../ddebiftool_extra_psol/',...
    '../../ddebiftool_utilities/',...
    '../../ddebiftool_extra_nmfm/');
clear;
close all
%#ok<*ASGLU,*NOPTS,*NASGU>

Definition of right-hand side

This time we vectorize the right-hand side in xx, but do not specify the derivatives analytically, relying on df_deriv. See demo1_funcs.html for details. The parameter vector has the order $[\kappa, \beta, a_{12}, a_{21}, \tau_1, \tau_2, \tau_s]$ .

neuron_sys_rhs=@(xx,par)[...
    -par(1)*xx(1,1,:)+par(2)*tanh(xx(1,4,:))+par(3)*tanh(xx(2,3,:));....
    -par(1)*xx(2,1,:)+par(2)*tanh(xx(2,4,:))+par(4)*tanh(xx(1,2,:))];
funcs=set_funcs(...
    'sys_rhs',neuron_sys_rhs,...
    'sys_tau',@()[5,6,7],...
    'x_vectorized',true);
ind_a21=4;  % used later for continuation
ind_taus=7; % used later for continuation
% general continuation parameters, kept in a cell list
parbd={'min_bound',[ind_a21,0],'max_bound',[ind_a21,4; ind_taus,10],...
    'max_step',[ind_a21,0.2; ind_taus,0.5]};

Steady state branches

The convenience function SetupStst can be used to define the initial piece of a steady-state branch. Its first arguments is funcs, the others are name-value pairs. Important parameters:

'parameter': row vector of initial parameters
'x': column vector of initial equilibrium
'contpar': index of continuation parameter (or vector of indices)
'step': initial step along branch (default 0.01)

All other name-value pairs are appended as fields to the structures in branch1 if their names match. Of course, the branch structure can also be manipulated manually afterwards. The subsequent continuation extends the branch in both directions up to the boundaries min_bound and max_bound.

[branch1,suc]=SetupStst(funcs,...
    'parameter',[1/2, -1, 1, 2.34, 0.2, 0.2, 1.5],'x',[0;0],...
    'contpar',ind_a21,'step',0.1,parbd{:});
branch1.method.continuation.plot=0; % don't plot prgress
[branch1,s,f,r]=br_contn(funcs,branch1,100);
branch1=br_rvers(branch1);
[branch1,s,f,r]=br_contn(funcs,branch1,100)

BR_CONTN warning: boundary hit.
BR_CONTN warning: boundary hit.
branch1 = 
       method: [1x1 struct]
    parameter: [1x1 struct]
        point: [1x24 struct]
s =
    14
f =
     0
r =
     0

Stability along branch

The convenience function GetStability recomputes the eigenvalues if not yet present and returns as its first output nunst the number of unstable eigenvalues for bifurcation detection. Its first argument is the branch structure for which stability information is required. We find the first point at which the number of unstable eigenvalues changes by 2.

branch1.method.stability.minimal_real_part=-2;
nunst=GetStability(branch1,'funcs',funcs);
indhopf=find(abs(diff(nunst))==2)

indhopf =
    19

Hopf continuation

Similar to SetupStst the convenience function SetupHopf creates the initial Hopf branch. Its first arguments are funcs, the branch along which the Hopf bifurcation was detected (here branch1), and the index of the point near which the Hopf bifurcation was detected. Important parameters:

'contpar': bifurcation parameters (vector of length >=2)
'dir': index of parameter, which is varied at initial step. The default is [], which means that only one point on the branch is computed. This is useful if one wants to correct only a single Hopf point.
'step': initial step along branch (default 1e-3)
'excudefreqs': list of frequencies that should be excluded (default []). The initial guess for the Hopf frequency is the complex conjugate pair closest to the imaginary axis, after one takes away a pair of eigenvalues for each frequency listed in excludefreqs.

All other name-value pairs can be used to replace fields in the structures of the Hopf branch. Otherwise, the output branch2 inherits all values from the input branch. The subsequent continuation extends the branch in both directions up to the boundaries min_bound and max_bound.

[branch2,suc]=SetupHopf(funcs,branch1,indhopf,'contpar',[ind_a21,ind_taus],...
    'dir',ind_taus,'step',0.1,'plot',1,parbd{:});
figure(1); clf;
[branch2,s,f,r]=br_contn(funcs,branch2,40);
branch2=br_rvers(branch2);
[branch2,s,f,r]=br_contn(funcs,branch2,20);
xlabel('a21');ylabel('\tau_s');

BR_CONTN warning: boundary hit.
BR_CONTN warning: boundary hit.

Figure: Continuation (predictions and corrections) of Hopf bifurcation

Predictions and corrections in the 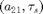$(a_{21},\tau_s)$ -plane after computation of a first branch of Hopf bifurcations.

Linear stability along Hopf curve

The function GetStability has a few additional outputs and inputs. The optional input exclude_trivial forces exclusion of trivial eigenvalues for bifurcations (for example the pair on the imaginary axis for the Hopf bifurcation) in the count of unstable eigenvalues. The second output (here dom) contains the dominant eigenvalue (that is, the closest to the imaginary axis, excluding the trivial eigenvalues). The third output (here triv_defect) is non-empty whenever exclude_trivial is true. It contains the defect between the known trivial eigenvalue (say. 1i*point.omega for Hopf points) and the value obtained in the stability calculation. The fourth output is the modified point array, now containing stability information.

[nunst_hopf,dom_hopf,triv_defect,branch2.point]=GetStability(branch2,...
    'funcs',funcs,'exclude_trivial',true);
% identify Takens-Bogdanov point (Hopf frequency crosses zero):
indTakens=find(diff(sign([branch2.point(:).omega]))~=0)

indTakens =
    37

Switch to second Hopf curve near double Hopf point

Repeat the Hopf continuation for the point on branch2 where another eigenvalue becomes unstable, again using SetupHopf, but now using 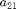$a_{21}$ as the parameter and initial step -0.05. Initially, we turn on residual printouts.

indhopf2=find(abs(diff(nunst_hopf))~=0,1,'first');
[branch3,suc]=SetupHopf(funcs,branch2,indhopf2,'contpar',[ind_a21,ind_taus],...
    'dir',ind_a21,'step',-0.05,'print_residual_info',1);
branch3.method.point.print_residual_info=0;
figure(1);
[branch3,s,f,r]=br_contn(funcs,branch3,100);
branch3=br_rvers(branch3);
[branch3,s,f,r]=br_contn(funcs,branch3,100);
xlabel('a21');ylabel('\tau_s');

it=1, res=0.0299965
it=2, res=0.00873529
it=3, res=4.20117e-05
it=4, res=1.54785e-08
it=5, res=8.85824e-16
it=1, res=0.0446972
it=2, res=0.00234939
it=3, res=1.40327e-05
it=4, res=5.80812e-10
it=5, res=5.55149e-16
BR_CONTN warning: boundary hit.
BR_CONTN warning: boundary hit.

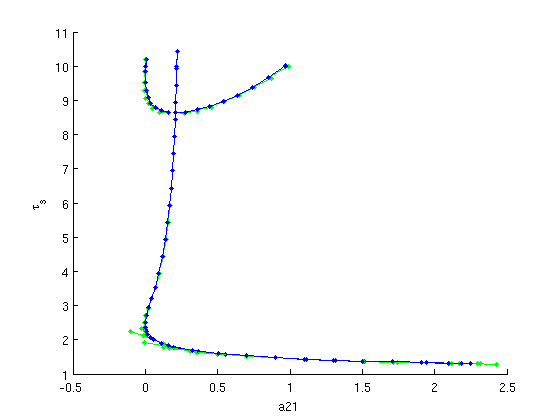
Figure: Continuation (predictions and corrections) of both Hopf bifurcations

Predictions and corrections in the 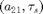$(a_{21},\tau_s)$ -plane after computation of second branch of Hopf bifurcations (superimposed on result of first Hopf bifurcation).

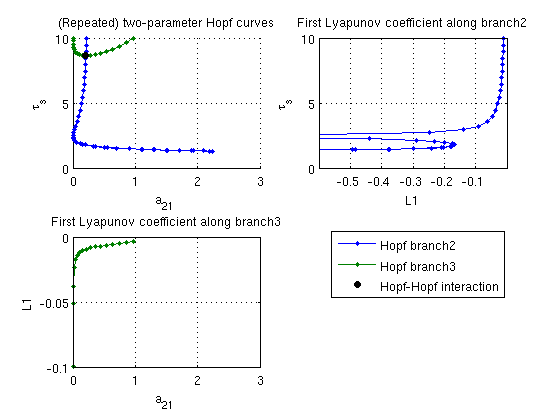
First Lyapunov coefficient L1 along both Hopf curves

After a Hopf curve is computed, HopfLyapunovCoefficients returns the first Lyapunov coefficient L1, which determines if the Hopf bifurcation is supercritical (L1<0) or subcritical (L1>0). Since L1 depends on 3rd-order derivatives of sys_rhs, the default finite-difference approximation df_mfderiv is susceptible to round-off errors. Hence, HopfLyapunovCoefficients returns 2 results, the second one using a lower-order approximation. The difference between both outputs gives an error estimate. The results show that both curves are supercritical.

[L1_br2,L1low_br2]=HopfLyapunovCoefficients(funcs,branch2);
[L1_br3,L1low_br3]=HopfLyapunovCoefficients(funcs,branch3);
fprintf('maximal L1 along branch2: %g\n',max(L1_br2))
fprintf('  max error of L1 along branch2: %g\n',max(abs(L1_br2-L1low_br2)));
fprintf('maximal L1 along branch3: %g\n',max(L1_br3))
fprintf('  max error of L1 along branch3: %g\n',max(abs(L1_br3-L1low_br3)));

maximal L1 along branch2: -0.0106291
  max error of L1 along branch2: 3.73912e-07
maximal L1 along branch3: -0.00375409
  max error of L1 along branch3: 5.38419e-10

Determine normal form of double Hopf point

Similar to the L1 coefficients, the normal form coefficients of a Hopf-Hopf point depend on 3rd-order derivatives of sys_rhs. Thus, the function computing the normal form returns two values, the difference of which is an error estimate. The output of HopfHopfNormalform is an entire point structure of kind 'hoho'. The field nmfm contains normal form coefficients. Other fields of interest are omega1 and omega2.

[hoho,hoho_low]=HopfHopfNormalform(funcs,branch2,indhopf2+(0:1));
fprintf(['Normal form coefficients of Hopf-Hopf point\n',...
    'at (a_21,tau_s)=(%g,%g) with omega1=%g, omega2=%g:\n'],...
    hoho.parameter(ind_a21),hoho.parameter(ind_taus),...
    hoho.omega1,hoho.omega2);
disp(hoho.nmfm);
fprintf('Error of normal form coefficients: %g\n',...
     norm(struct2array(hoho.nmfm)-struct2array(hoho_low.nmfm),'inf'));

Bisection residual: res(1)=0.00376645, res(2)=-0.0026432
Bisection: new residual=0.000699409
Bisection: new residual=-0.000935621
Bisection: new residual=-0.000109275
Bisection: new residual=0.000297246
Bisection: new residual=9.45336e-05
Bisection: new residual=-7.2333e-06
Bisection: new residual=4.36845e-05
Bisection: new residual=1.82342e-05
Bisection: new residual=5.50258e-06
Normal form coefficients of Hopf-Hopf point
at (a_21,tau_s)=(0.2073,8.6345) with omega1=0.328701, omega2=0.915667:
    g2100: -0.0042 - 0.0013i
    g1011: -0.0091 - 0.0029i
    g1110: -0.0158 + 0.0053i
    g0021: -0.0086 + 0.0029i
    theta: 1.0604
    delta: 3.7721
Error of normal form coefficients: 1.9448e-07

Two-parameter bifurcation diagram of Hopf bifurcations

with L1 coefficients

a21_br2 =arrayfun(@(x)x.parameter(ind_a21), branch2.point);
taus_br2=arrayfun(@(x)x.parameter(ind_taus),branch2.point);
a21_br3 =arrayfun(@(x)x.parameter(ind_a21), branch3.point);
taus_br3=arrayfun(@(x)x.parameter(ind_taus),branch3.point);
figure(3);
subplot(2,2,1);
hold on
ph_br2=plot(a21_br2,taus_br2,'.-');
ph_br3=plot(a21_br3,taus_br3,'.-','color',[0,0.5,0]);
ph_hoho=plot(hoho.parameter(ind_a21),hoho.parameter(ind_taus),...
    'ko','markerfacecolor','k');
lg_handles=[ph_br2,ph_br3,ph_hoho];
lg_texts={'Hopf branch2','Hopf branch3','Hopf-Hopf interaction'};
lh=legend(lg_handles,lg_texts);
lc=get(lh,'position');
set(lh,'position',[0.9-lc(3),0.45-lc(4),lc(3:4)])
a21lim=get(gca,'xlim');
tauslim=get(gca,'ylim');
grid on
xlabel('a_{21}');
ylabel('\tau_s');
title('(Repeated) two-parameter Hopf curves');
subplot(2,2,2);
plot(L1_br2,taus_br2,'.-');
set(gca,'ylim',tauslim,'xlim',[-0.6,0]);
grid on
ylabel('\tau_s');
xlabel('L1');
title('First Lyapunov coefficient along branch2');
subplot(2,2,3);
plot(a21_br3,L1_br3,'.-','color',[0,0.5,0]);
set(gca,'ylim',[-0.1,0],'xlim',a21lim);
grid on
xlabel('a_{21}');
ylabel('L1');
title('First Lyapunov coefficient along branch3');

Periodic orbit continuation

The convenience function SetupPsol creates the initial branch of periodic orbits. Its first arguments are funcs, the Hopf or steady-state branch along which a Hopf bifurcation was detected, and the index of the Hopf point along the branch where one wants to branch off point. Important additional parameters:

'radius': amplitude of initial periodic orbit (default 1e-3),
'contpar': index of continuation parameter (otherwise taken from input branch),
'degree': degree of collocation polynomials (default 3)
'intervals': number of collocation intervals (default 20)
'hopfcorrection': apply Newton iteration to find correct Hopf point before branching off. This is recommended if the point provided is not of kind 'hopf'.

All other parameters are passed on to the resulting output branch of periodic orbits (here branch4). The parameter field of the output branch is inherited from the input branch (before optional parameters are taken into account).

[branch4,suc]=SetupPsol(funcs,branch1,indhopf,'degree',4,'intervals',40);
branch4.parameter.max_step(1,:)=[ind_a21,0.1];
figure(2); clf;
[branch4,s,f,r]=br_contn(funcs,branch4,50);
% look at the period along the branch:
a21_per=arrayfun(@(x)x.parameter(4),branch4.point);
periods=[branch4.point.period];
figure(4); clf;
plot(a21_per,periods,'b.-');
xlabel('a21');
ylabel('period');

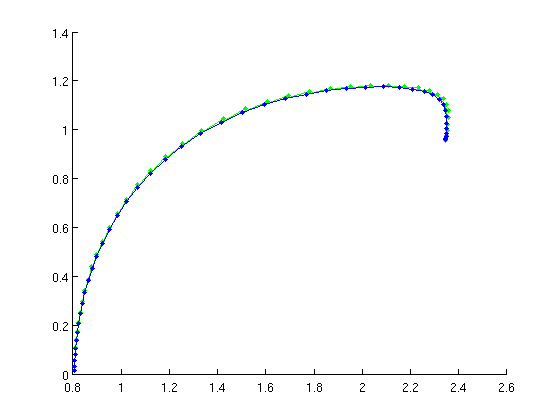
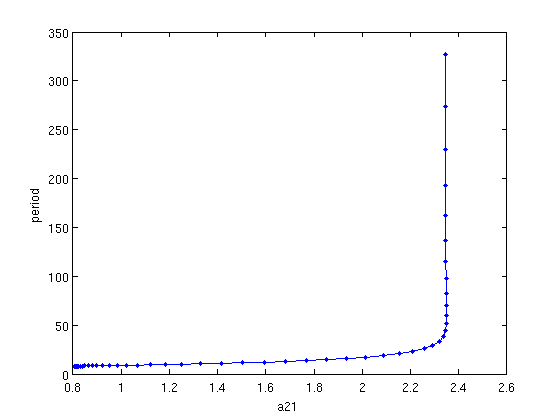
Figure: family of periodic orbits

Branch of periodic solutions emanating from a Hopf point. The branch turns at the far right.

Floquet multipliers of periodic orbits

The function GetStability can be used for periodic orbits as well.

[nunst_per,dom,trivdef_per,branch4.point]=GetStability(branch4,...
    'funcs',funcs,'exclude_trivial',true);
fprintf('Loss of stability at point %d\n',find(nunst_per,1,'first'));

Warning: Matrix is close to singular or badly scaled. Results may be
inaccurate. RCOND =  2.009522e-18. 
Loss of stability at point 42

Continuation of folds of periodic orbits

See demo1_POfold.html for extensive explanation. find inital approximate fold

[dummy,indmax]=max(arrayfun(@(x)x.parameter(ind_a21),branch4.point));
% initialize problem functions and branch
[foldfuncs,branch5]=SetupPOfold(funcs,branch4,indmax,'contpar',[ind_a21,ind_taus],...
    'dir',ind_taus,'print_residual_info',1,'step',0.01,'plot_measure',[],...
    parbd{:});
% do not print residuals along branch
branch5.method.point.print_residual_info=0;
% continue branch
figure(1);
branch5=br_contn(foldfuncs,branch5,100);
xlabel('a21');ylabel('tau_s');
title('Continuation of fold of periodic orbits');
% get the profile of the periodic orbits and compute their Floquet
% multipliers and stability
pf_orbits=foldfuncs.get_comp(branch5.point,'solution');
[nunst_pf,dom,triv_defect,pf_orbits]=GetStability(pf_orbits,'funcs',funcs,...
    'exclude_trivial',true,'locate_trivial',@(x)[1,1]);
fprintf('max number of unstable Floquet multipliers: %d\n',max(nunst_pf));
% plot the defect of the trivial Floquet multiplier as computed by
% GetStability
n_orbits=length(pf_orbits);
figure(5);
a21_pfold=arrayfun(@(x)x.parameter(ind_a21),branch5.point);
taus_pfold=arrayfun(@(x)x.parameter(ind_taus),branch5.point);
plot(1:n_orbits,triv_defect);
xlabel('point number');
ylabel('defect of trivial Floquet multiplier');
title('Comparison of Floquet multiplier computation with extended system');

it=1, res=0.000616502
it=2, res=0.00872625
it=3, res=0.000152002
it=4, res=5.54573e-08
it=5, res=2.30907e-10
it=1, res=0.000362284
it=2, res=2.0391e-09
it=1, res=0.0699005
it=2, res=0.0147462
it=3, res=6.48903e-06
it=4, res=3.38845e-10
it=1, res=0.000255849
it=2, res=6.59513e-10
BR_CONTN warning: boundary hit.
max number of unstable Floquet multipliers: 0

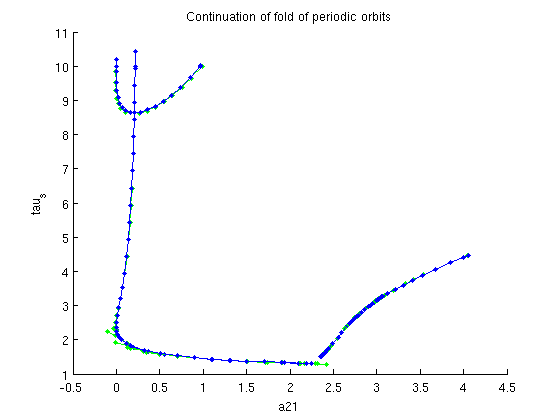
Add fold of periodic orbits to Two-parameter bifurcation diagram

figure(3);
subplot(2,2,1);
hold on
ph_pfold=plot(a21_pfold,taus_pfold,'r.-');
lg_handles(end+1)=ph_pfold;
lg_texts{end+1}='Fold of periodic orbits';
lh=legend(lg_handles,lg_texts);
lc=get(lh,'position');
set(lh,'position',[0.9-lc(3),0.45-lc(4),lc(3:4)])
a21lim=get(gca,'xlim');
subplot(2,2,3);
set(gca,'xlim',a21lim);

save data:

save('demo1_simple_results.mat');